Page 159 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 10: Correlation: Scatter Diagram Method, Karl Pearson's Coefficient of Correlation

Notes
1
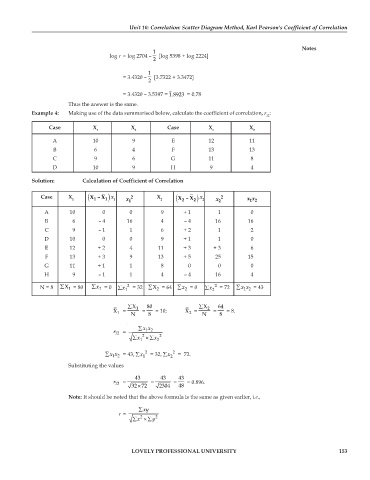
log r = log 2704 – [log 5398 + log 2224]
2
1
= 3.4320 – [3.7322 + 3.3472]
2

= 3.4320 – 3.5397 = 1.8923 = 0.78
Thus the answer is the same.
Example 4: Making use of the data summarised below, calculate the coefficient of correlation, r :
12
Case X X Case X X
1 2 1 2
A 10 9 E 12 11
B 6 4 F 13 13
C 9 6 G 11 8
D 10 9 H 9 4

Solution: Calculation of Coefficient of Correlation

Case X ( X– X ) x 2 X ( x 2
12
1 1 1 1 x 1 2 2 2 ) X– X 2 x 2 xx
A 10 0 0 9 + 1 1 0
B 6 – 4 16 4 – 4 16 16
C 9 – 1 1 6 + 2 1 2
D 10 0 0 9 + 1 1 0
E 12 + 2 4 11 + 3 + 3 6
F 13 + 3 9 13 + 5 25 15
G 11 + 1 1 8 0 0 0
H 9 – 1 1 4 – 4 16 4

N = 8 ∑X = 80 ∑x = 0 ∑x 1 2 = 32 ∑X = 64 ∑x = 0 ∑x 2 2 = 72 ∑xx = 43
12
1
1
2
2
∑X 80 ∑X 64
X 1 = N 1 = 8 = 10; X = N 2 = 8 = 8.
2
∑xx
r 12 = 12
∑ 1 2 ×x ∑ x 2 2
∑xx = 43, ∑x 1 2 = 32, ∑x 2 2 = 72.
12
Substituting the values
43 43 43
r 12 = 32 ×72 = 2304 = 48 = 0.896.

Note: It should be noted that the above formula is the same as given earlier, i.e.,

∑xy
r =
∑ 2 ×x ∑ y 2

LOVELY PROFESSIONAL UNIVERSITY 153