Page 141 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

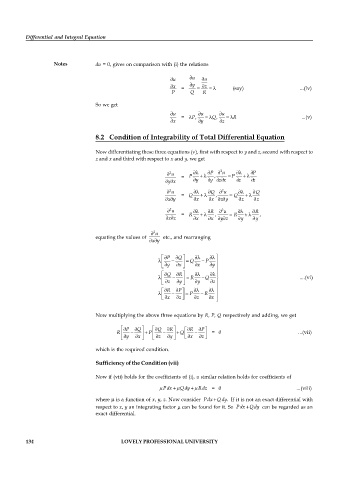
Notes du = 0, gives on comparison with (i) the relations

u u u
x = y z (say) ...(iv)
P Q R
So we get
u u u
= , P Q , R ...(v)
x y z

8.2 Condition of Integrability of Total Differential Equation

Now differentiating these three equations (v), first with respect to y and z, second with respect to
z and x and third with respect to x and y, we get

2 P 2 u P
u P , P
=
y x y y z x z z
2 2
u Q u Q
= Q , Q
x y x x z y z z
2 2
u R u R
= R , R ,
x z x x y z y y
2 u
equating the values of etc., and rearranging
x y

P Q
Q P
y x x y
Q R
R Q ....(vi)
z y y z
R P
P R
x z z x

Now multiplying the above three equations by R, P, Q respectively and adding, we get

P Q Q R R P
R P Q = 0 ...(vii)
y x z y x z
which is the required condition.

Sufficiency of the Condition (vii)

Now if (vii) holds for the coefficients of (i), a similar relation holds for coefficients of

µPdx µQdy µRdz = 0 ...(viii)
.
where is a function of x, y, z. Now consider Pdx Qdy If it is not an exact differential with
respect to x, y an integrating factor can be found for it. So Pdx Qdy can be regarded as an
exact differential.

134 LOVELY PROFESSIONAL UNIVERSITY