Page 144 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 8: Total Differential Equations, Simultaneous Equations

Notes
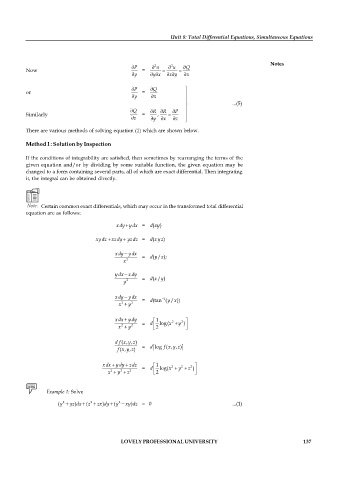
P 2 u 2 u Q
Now =
y y x x y x
P Q
or =
y x
...(5)
Q R R P
Similarly = ,
z y x z
There are various methods of solving equation (1) which are shown below.

Method I : Solution by Inspection

If the conditions of integrability are satisfied, then sometimes by rearranging the terms of the
given equation and/or by dividing by some suitable function, the given equation may be
changed to a form containing several parts, all of which are exact differential. Then integrating
it, the integral can be obtained directly.

Note: Certain common exact differentials, which may occur in the transformed total differential
equation are as follows:

xdy ydx = d (xy )

(x
xydz xzdy yzdz = d yz )
xdy y dx
x
= d ( / );
y
x 2
y dx xdy
y
x
y 2 = d ( / )
xdy y dx 1
= d (tan ( / ))
y
x
x 2 y 2
xdx y dy 1 2 2
x 2 y 2 = d 2 log(x y )

z
d f ( , , )
y
x
z
x
y
= d log ( , , )
f
y
x
z
f ( , , )
xdx y dy zdz 1 2 2 2
= d log(x y z )
x 2 y 2 z 2 2
Example 1: Solve
(y 2 yz )dx (z 2 zx )dy (y 2 xy )dz = 0 ...(1)

LOVELY PROFESSIONAL UNIVERSITY 137