Page 142 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 8: Total Differential Equations, Simultaneous Equations

Notes
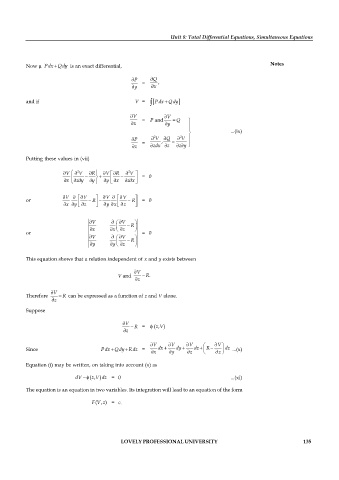
Now Pdx Qdy is an exact differential,
P Q
= ,
y x

and if V = Pdx Qdy

V V
= P and Q
x y
...(ix)
2 2
P V Q V
= ,
z zdx z z y
Putting these values in (vii)

V 2 V R V R 2 V
= 0
x z y y y x z x

V V V V
or R R = 0
x y z y x z

V V
R
x x z
or = 0
V V
R
y y z
This equation shows that a relation independent of x and y exists between

V
V and . R
z

V
Therefore R can be expressed as a function of z and V alone.
z
Suppose
V
V
z
R = ( , )
z
V V V V
Since Pdx Qdy Rdz = dx dy dz R dz ...(x)
x y z z

Equation (i) may be written, on taking into account (x) as
dV ( , )dz = 0 ...(xi)
z
V
The equation is an equation in two variables. Its integration will lead to an equation of the form

V
F ( , ) = c.
z

LOVELY PROFESSIONAL UNIVERSITY 135