Page 157 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

Notes
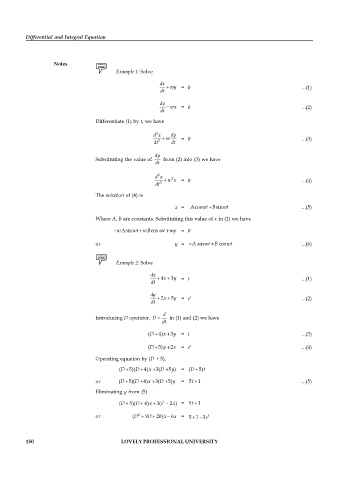
Example 1: Solve

dx
wy = 0 ...(1)
dt
dy
wx = 0 ...(2)
dt
Differentiate (1) by t, we have

2
d x w dy = 0 ...(3)
dt 2 dt

dy
Substituting the value of from (2) into (3) we have
dt
2
d x w x = 0
2
dt 2 ...(4)
The solution of (4) is

x = A cos wt B sin wt ...(5)

Where A, B are constants. Substituting this value of x in (1) we have
wA sin wt wB cos wt wy = 0

or y = A sin wt B cos wt ...(6)

Example 2: Solve

dx
4x 3y = t ...(1)
dt
dy
2x 5y = e t ...(2)
dt
d
Introducing D operator, D in (1) and (2) we have
dt

(D 4)x 3y = t ...(3)

(D 5)y 2x = e t ...(4)
Operating equation by (D + 5),
y
(D 5)(D 4)x 3(D 5 ) = (D 5)t
or (D 5)(D 4)x 3(D 5)y = 5t 1 ...(5)
Eliminating y from (5)

(D 5)(D 4)x 3(e t 2 ) = 5t 1
x
or (D 2 9D 20)x 6x = 5 1 3e t

150 LOVELY PROFESSIONAL UNIVERSITY