Page 159 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

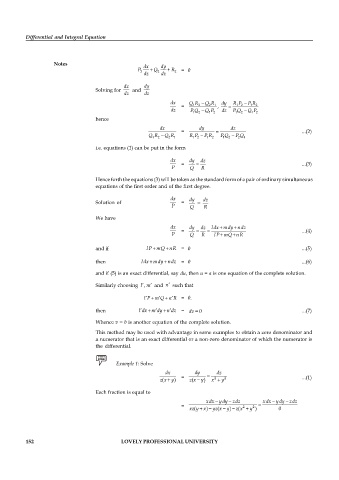
Notes dx dy
P 2 Q 2 R 2 = 0
dz dz
dx dy
Solving for and
dz dz
dx Q R Q R dy R P P R
= 1 2 2 1 , 1 2 1 2
dz P Q Q P dz P Q Q P
1 2 1 2 1 2 1 2
hence
dx dy dz
= ...(2)
Q R Q R R P P R P Q P Q
1 2 2 1 1 2 1 2 1 2 2 1
i.e. equations (1) can be put in the form

dx dy dz
= ...(3)
P Q R
Hence forth the equations (3) will be taken as the standard form of a pair of ordinary simultaneous
equations of the first order and of the first degree.

dx dy dz
Solution of =
P Q R
We have
dx dy dz ldx mdy ndz
= ...(4)
P Q R lP mQ nR

and if lP mQ nR = 0 ...(5)

then ldx mdy ndz = 0 ...(6)
and if (5) is an exact differential, say du, then u = a is one equation of the complete solution.

Similarly choosing ,l m and n such that

l P m Q n R = 0.

then l dx m dy n dz = dv 0 ...(7)
Whence v = b is another equation of the complete solution.
This method may be used with advantage in some examples to obtain a zero denominator and
a numerator that is an exact differential or a non-zero denominator of which the numerator is
the differential.

Example 1: Solve
dx dy dz
= 2 2 ...(1)
( z x y ) ( z x y ) x y

Each fraction is equal to
xdx y dy zdz xdx y dy zdz
= 2 2
xz (y x ) yz (x y ) z (x y ) 0

152 LOVELY PROFESSIONAL UNIVERSITY