Page 277 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Differential and Integral Equation

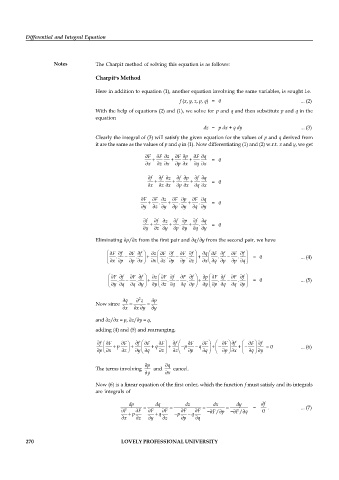
Notes The Charpit method of solving this equation is as follows:
Charpit s Method

Here in addition to equation (1), another equation involving the same variables, is sought i.e.
f (x, y, z, p, q) = 0 ... (2)
With the help of equations (2) and (1), we solve for p and q and then substitute p and q in the
equation

dz = p dx + q dy ... (3)
Clearly the integral of (3) will satisfy the given equation for the values of p and q derived from
it are the same as the values of p and q in (1). Now differentiating (1) and (2) w.r.t. x and y, we get

F F z F p F q
= 0
x z x p x q x
f f z f p f q
= 0
x z x p x q x
F F z F p F q
. . . = 0
y z y p y q y

f f z f p f q
. . . = 0
y z y p y q y
Eliminating p/ x from the first pair and q/ y from the second pair, we have

F f F f z F f F f q F f F f
. . . . . . = 0 ... (4)
x p p x x z p p z x q p p q

F f F f z F f F f p F f F f
. . . . . . = 0 ... (5)
y q q y y z q q p y p q q p

q 2 z p
Now since
x x y y

and z/ x = p, z/ y = q,
adding (4) and (5) and rearranging,

f F F f F F f F F F f F f
p q p q = 0 ... (6)
p x z y q z z p q p x q y

p q
The terms involving and cancel.
y x
Now (6) is a linear equation of the first order, which the function f must satisfy and its integrals
are integrals of

dp dq dz dx dy df
= . ... (7)
F F F F F F F / p F / q 0
p q p q
x z y z p q

270 LOVELY PROFESSIONAL UNIVERSITY