Page 28 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION
P. 28
,
Unit 1: Bessel s Functions
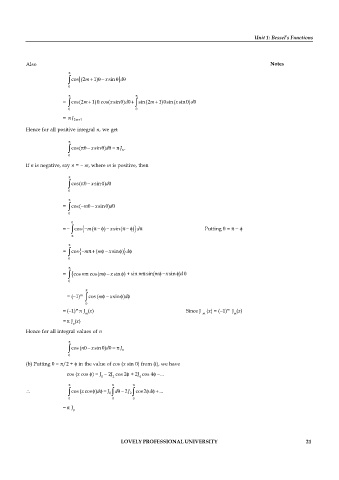
Also Notes
cos (2m 1) x sin d
0
x
x
= cos(2m 1) .cos( sin )d sin(2m 1) sin( sin )d
0 0
= J 2m 1
Hence for all positive integral n, we get
cos( x sin )d J .
n
0
If n is negative, say n = m, where m is positive, then
cos( x sin )d
0
= cos( m x sin )d
0
0
= cos m ( ) x sin( ) d Putting =
= cos m (m x sin ) d
0
= cos m cos(m x sin ) + sin m sin(m x sin )d
0
m
= ( 1) cos (m x sin )d
0
m
= ( 1) m J (x) Since J (x) = ( 1) J (x)
m m m
= J (x)
n
Hence for all integral values of n
cos (n x sin )d J n
0
(b) Putting = /2 + in the value of cos (x sin ) from (i), we have
cos (x cos ) = J 2J cos 2 + 2J cos 4 ...
0 2 4
x
cos ( cos )d J 0 d 2J 2 cos2 d ...
0 0 0
= J
0
LOVELY PROFESSIONAL UNIVERSITY 21