Page 101 - DMTH202_BASIC_MATHEMATICS_II
P. 101
Basic Mathematics-II
Notes 7. In definite practice the integrals are frequently mentioned not as 0 to x but as from a lower
limit to a ................................... limit corresponding to the area preferred.
8. The ................................... of the integrals can be revealed by the estimation method sketched
to be equal to the area under the curve.
9. The integrals exhibit that the area under the curve as displayed by the integral is in fact the
antiderivative with the ................................... limits.
10. Make the trapezoids minute enough and they get somewhat ................................... to rectangles.
State whether the following statements are true or false:
11. This estimation approach can be carried to superior correctness by making slighter and
slighter trapezoids.
12. The integral is scrutinized as the area produced by summing a finite number of rectangles
of infinitely minute width.
7.2 Area within Two or more Curves
The technique for identifying the area within two or more curves is an imperative application of
integral calculus. There are three major steps to this procedure. They are:
1. Graph the two or more equations.
2. Find out the points of intersection.
3. Set up and assess the definite integral.
In the following examples, this process is demonstrated.
Did u know? The technique for identifying the area within two or more curves allows us
identify the area of irregular shapes by assessing the definite integral.
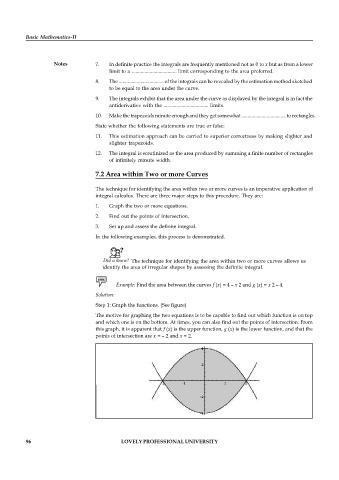
Example: Find the area between the curves f (x) = 4 – x 2 and g (x) = x 2 – 4.
Solution:
Step 1: Graph the functions. (See figure)
The motive for graphing the two equations is to be capable to find out which function is on top
and which one is on the bottom. At times, you can also find out the points of intersection. From
this graph, it is apparent that f (x) is the upper function, g (x) is the lower function, and that the
points of intersection are x = – 2 and x = 2.
96 LOVELY PROFESSIONAL UNIVERSITY