Page 156 - DCAP601_SIMULATION_AND_MODELING

Simulation and Modelling

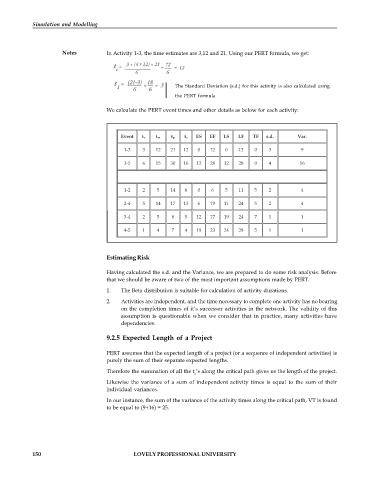
Notes In Activity 1-3, the time estimates are 3,12 and 21. Using our PERT formula, we get:

The Standard Deviation (s.d.) for this activity is also calculated using
the PERT formula

We calculate the PERT event times and other details as below for each activity:

Event to tm tp te ES EF LS LF TF s.d. Var.
1-3 3 12 21 12 0 12 0 12 0 3 9

3-5 6 15 30 16 12 28 12 28 0 4 16

1-2 2 5 14 6 0 6 5 11 5 2 4

2-4 5 14 17 13 6 19 11 24 5 2 4
3-4 2 5 8 5 12 17 19 24 7 1 1

4-5 1 4 7 4 19 23 24 28 5 1 1

Estimating Risk

Having calculated the s.d. and the Variance, we are prepared to do some risk analysis. Before
that we should be aware of two of the most important assumptions made by PERT.

1. The Beta distribution is suitable for calculation of activity durations.
2. Activities are independent, and the time necessary to complete one activity has no bearing
on the completion times of it’s successor activities in the network. The validity of this
assumption is questionable when we consider that in practice, many activities have
dependencies.

9.2.5 Expected Length of a Project

PERT assumes that the expected length of a project (or a sequence of independent activities) is
purely the sum of their separate expected lengths.
Therefore the summation of all the t ’s along the critical path gives us the length of the project.
e
Likewise the variance of a sum of independent activity times is equal to the sum of their
individual variances.
In our instance, the sum of the variance of the activity times along the critical path, VT is found
to be equal to (9+16) = 25.

150 LOVELY PROFESSIONAL UNIVERSITY