Page 270 - DMGT403_ACCOUNTING_FOR_MANAGERS

Unit 12: Marginal Costing and Profit Planning

Notes
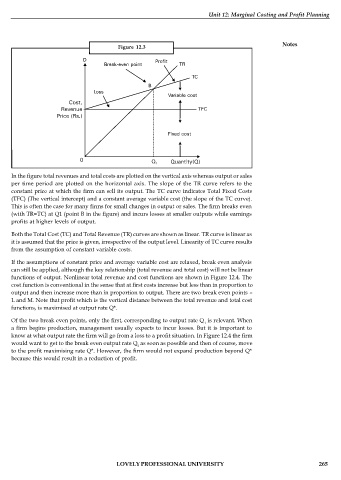
Figure 12.3

In the figure total revenues and total costs are plotted on the vertical axis whereas output or sales
per time period are plotted on the horizontal axis. The slope of the TR curve refers to the
constant price at which the firm can sell its output. The TC curve indicates Total Fixed Costs
(TFC) (The vertical intercept) and a constant average variable cost (the slope of the TC curve).
This is often the case for many firms for small changes in output or sales. The firm breaks even
(with TR=TC) at Q1 (point B in the figure) and incurs losses at smaller outputs while earnings
profits at higher levels of output.

Both the Total Cost (TC) and Total Revenue (TR) curves are shown as linear. TR curve is linear as
it is assumed that the price is given, irrespective of the output level. Linearity of TC curve results
from the assumption of constant variable costs.
If the assumptions of constant price and average variable cost are relaxed, break even analysis
can still be applied, although the key relationship (total revenue and total cost) will not be linear
functions of output. Nonlinear total revenue and cost functions are shown in Figure 12.4. The
cost function is conventional in the sense that at first costs increase but less than in proportion to
output and then increase more than in proportion to output. There are two break even points –
L and M. Note that profit which is the vertical distance between the total revenue and total cost
functions, is maximised at output rate Q*.
Of the two break even points, only the first, corresponding to output rate Q is relevant. When
1
a firm begins production, management usually expects to incur losses. But it is important to
know at what output rate the firm will go from a loss to a profit situation. In Figure 12.4 the firm
would want to get to the break even output rate Q as soon as possible and then of course, move
1
to the profit maximising rate Q*. However, the firm would not expand production beyond Q*
because this would result in a reduction of profit.

LOVELY PROFESSIONAL UNIVERSITY 265