Page 105 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 7: Mean Deviation and Standard Deviation

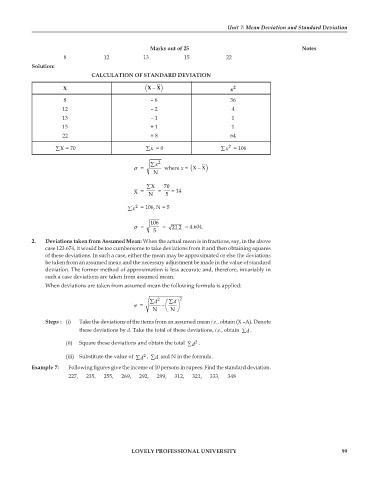
Marks out of 25 Notes
8 12 13 15 22
Solution:
CALCULATION OF STANDARD DEVIATION

X ( X ) X − x 2
8 – 6 36
12 – 2 4
13 – 1 1
15 + 1 1
22 + 8 64

2
∑X = 70 ∑x = 0 ∑x = 106
∑x 2
σ = where x = ( XX ) −
N

∑X 70
X = N = 5 = 14

∑x 2 = 106, N = 5

106
σ = = 21.2 = 4.604.
5
2. Deviations taken from Assumed Mean: When the actual mean is in fractions, say, in the above
case 123.674, it would be too cumbersome to take deviations from it and then obtaining squares
of these deviations. In such a case, either the mean may be approximated or else the deviations
be taken from an assumed mean and the necessary adjustment be made in the value of standard
deviation. The former method of approximation is less accurate and, therefore, invariably in
such a case deviations are taken from assumed mean.
When deviations are taken from assumed mean the following formula is applied:

∑ 2 ⎛ ∑d d ⎞ 2
σ = − ⎜ ⎟
⎝ N ⎠ N

Steps : (i) Take the deviations of the items from an assumed mean i.e., obtain (X –A). Denote
these deviations by d. Take the total of these deviations, i.e., obtain ∑d .

(ii) Square these deviations and obtain the total ∑d 2 .

(iii) Substitute the value of ∑d 2 , ∑d and N in the formula.
Example 7: Following figures give the income of 10 persons in rupees. Find the standard deviation.
227, 235, 255, 269, 292, 299, 312, 321, 333, 348

LOVELY PROFESSIONAL UNIVERSITY 99