Page 110 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Statistical Methods in Economics

Notes
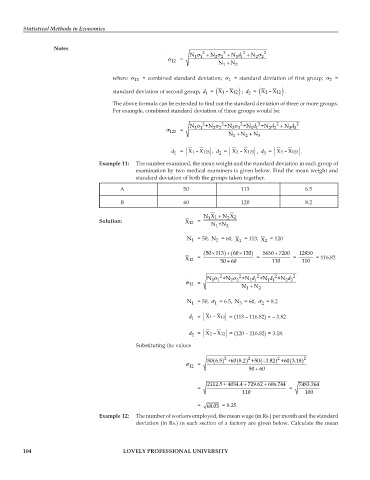
N σ 2 + N σ 2 + N d 2 + N σ 2
σ 12 = 1 1 22 1 N 1 1 22
N +
2
where σ 12 = combined standard deviation; σ = standard deviation of first group; σ =
1
2
standard deviation of second group; d = ( 1 1 12 ; d = ( ) X– X 2 2 12 .
) X– X
The above formula can be extended to find out the standard deviation of three or more groups.
For example, combined standard deviation of three groups would be:

N σ 2 σ +N 2 +N σ 2 +N d 2 +N d 2 + N d 2
σ 123 = 11 2 2 3 3 1 1 2 2 3 3
1 + N 2 + N N 3

d 1 = X– X 123 , d = X– X 123 , d = X– X 123 .
2
1
3
3
2
Example 11: The number examined, the mean weight and the standard deviation in each group of
examination by two medical examiners is given below. Find the mean weight and
standard deviation of both the groups taken together.
A 50 113 6.5
B 60 120 8.2

NX 1 + N X 2
Solution: X 12 = 1 N+N 2 2
1
N 1 = 50, N = 60, X = 113, X = 120
2
1
2
( )50 113 + (× × )60 120 5650 + 7200 12850
X 12 = 50 + 60 = 110 = 110 = 116.82

N σ 2 σ +N 2 +N d 2 +N d 2 +N d 2
σ 12 = 1 1 2 2 N 1 11 2 11 2 2
+ N

N 1 = 50, σ = 6.5, N = 60, σ = 8.2
2
1
3
d 1 = X– X 12 = (113 – 116.82) = – 3.82
1
d 2 = X– X 12 = (120 – 116.82) = 3.18.
2
Substituting the values

2
( 50 )6.5 +60 ( )8.2 2 ( +50 − ) 3.82 2 ( +60 3.18 ) 2
σ 12 =
+
50 60
+
2112.5 + + 4034.4 729.62 606.744 7483.264
= =
110 100
= 68.03 = 8.25.
Example 12: The number of workers employed, the mean wage (in Rs.) per month and the standard
deviation (in Rs.) in each section of a factory are given below. Calculate the mean

104 LOVELY PROFESSIONAL UNIVERSITY