Page 113 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 7: Mean Deviation and Standard Deviation

said to be less variable or more consistent, more uniform more stable or more homogeneous. Coefficient Notes
of variation is denoted by the symbol C.V. and is obtained as follows:
σ
Coefficient of variation or C.V. = × 100 .
X
It may be pointed out that although any measure of dispersion can be used in conjunction with any
average in computing relative dispersion, statisticians, in fact, almost always use the standard deviation
as the measure of dispersion and the arithmetic mean as the average. When the relative dispersion is
stated in terms of the arithmetic mean and the standard deviation, the resulting percentage is known
as the coefficient of variation of coefficient of variability.
A distinction is sometimes made between coefficient of variation and coefficient of standard deviation.
⎛ σ ⎞
The former is always a percentage, the latter is just the ratio of standard deviation to mean, i.e., ⎜ ⎟ .
⎝ X ⎠
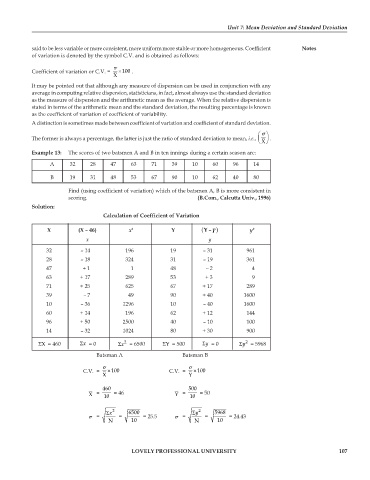
Example 13: The scores of two batsmen A and B in ten innings during a certain season are:
A 32 28 47 63 71 39 10 60 96 14

B 19 31 48 53 67 90 10 62 40 80

Find (using coefficient of variation) which of the batsmen A, B is more consistent in
scoring. (B.Com., Calcutta Univ., 1996)
Solution:
Calculation of Coefficient of Variation

X (X – 46) x 2 Y (Y– γ ) y 2
x y
32 – 14 196 19 – 31 961
28 – 18 324 31 – 19 361
47 + 1 1 48 – 2 4
63 + 17 289 53 + 3 9
71 + 25 625 67 + 17 289
39 – 7 49 90 + 40 1600
10 – 36 1296 10 – 40 1600
60 + 14 196 62 + 12 144
96 + 50 2500 40 – 10 100
14 – 32 1024 80 + 30 900
2
2
ΣX = 460 Σx = 0 Σx = 6500 ΣY = 500 Σy = 0 Σy = 5968
Batsman A Batsman B
σ σ
C.V. = × 100 C.V. = × 100
X Y

460 500
X = 10 = 46 Y = 10 = 50

Σx 2 6500 Σ y 2 5968
σ = = = 25.5 σ = = = 24.43
N 10 N 10

LOVELY PROFESSIONAL UNIVERSITY 107