Page 111 - DECO504_STATISTICAL_METHODS_IN_ECONOMICS_ENGLISH

Unit 7: Mean Deviation and Standard Deviation

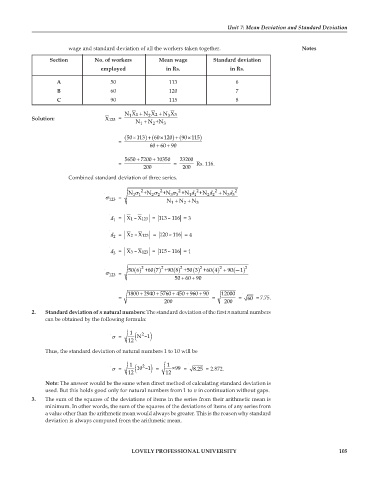
wage and standard deviation of all the workers taken together. Notes

Section No. of workers Mean wage Standard deviation
employed in Rs. in Rs.
A 50 113 6
B 60 120 7
C 90 115 8

NX 1 + N X 2 + N X 3
2
Solution: X 123 = 1 N + N +N 3 3
2
1
( )50 113 + (× )60 120 + (× × )90 115
=
+
+ 60 60 90
+
+ 5650 7200 10350 23200
= = Rs. 116.
200 200
Combined standard deviation of three series.

σ
σ
N 11 2 2 2 2 +N 3 3 2 +N d 2 +N d 2 + N d 2
σ +N
3 3
2 2
1 1
σ 123 =
1 + N 2 + N N 3
d = X– X 123 = 113 – 116 = 3
1
1
d 2 = X– X 123 = 120 – 116 = 4
2
d 3 = X– X 123 = 115 – 116 = 1
3
2
( ) +90 8 +50 3 +60
σ 123 = 50 () 6 +60 7 2 ( ) 2 () 2 ( ) +4 2 90 (—1 ) 2
+ 50 60 90
+
+
+
+
+
+ 1800 2940 5760 450 960 90 12000
= = = 60 = 7.75.
200 200
2. Standard deviation of n natural numbers: The standard deviation of the first n natural numbers
can be obtained by the following formula:
1
σ = ( 2 ) N–1
12
Thus, the standard deviation of natural numbers 1 to 10 will be
1 ( 2 1
σ = ) 10 –1 = ×99 = 8.25 = 2.872.
12 12
Note: The answer would be the same when direct method of calculating standard deviation is
used. But this holds good only for natural numbers from 1 to n in continuation without gaps.
3. The sum of the squares of the deviations of items in the series from their arithmetic mean is
minimum. In other words, the sum of the squares of the deviations of items of any series from
a value other than the arithmetic mean would always be greater. This is the reason why standard
deviation is always computed from the arithmetic mean.

LOVELY PROFESSIONAL UNIVERSITY 105