Page 258 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 17: Lagrange’s Methods for Solving Partial Differential Equations

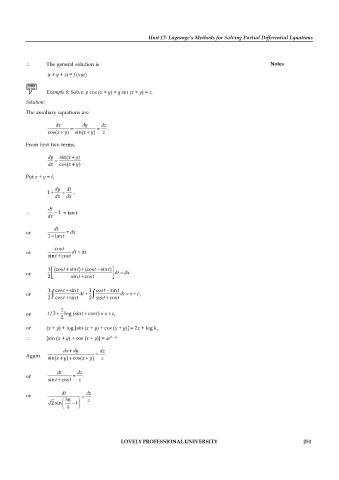
The general solution is Notes
(x + y + z) = f (xyz)

Example 8: Solve: p cos (x + y) + q sin (x + y) = z.
Solution:
The auxiliary equations are

dx dy dz
.
cos(x y ) sin(x y ) z
From first two terms,

dy sin(x y )
dx cos(x y ) .
Put x + y = t,

dy dt
1 ,
dx dx
dt
1 = tan t
dx

dt
or dx
1 tant
cost
or dt dx
sint cost
t
1 (cost sin ) (cost sin )
t
or dt dx
2 sint cost
1 cost sint 1 cost sint
or dt dt x c 1
2 cost sint 2 sint cost

1
t
or t /2 log (sint cos ) x c 1
2
or (x + y) + log [sin (x + y) + cos (x + y)] = 2x + log k .
1
[sin (x + y) + cos (x + y)] = ae x y
dx dy dz
Again .
sin(x y ) cos(x y ) z
dt dz
or .
sint cost z

dt dz
or
3 z
2 sin t
4

LOVELY PROFESSIONAL UNIVERSITY 251