Page 260 - DMTH504_DIFFERENTIAL_AND_INTEGRAL_EQUATION

Unit 17: Lagrange’s Methods for Solving Partial Differential Equations

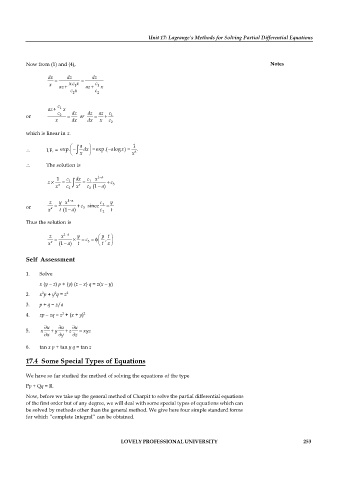
Now from (1) and (4), Notes
dx dz dz
x . x c x c 1
1
az az x
c x c 2
2
c
az 1 x
c dz dz az c
or 2 or 1
x dx dx x c 2
which is linear in z.

a 1
x
I.F. = exp. dx exp.( a log ) a .
x x
The solution is

1 c dx c x 1 a
z 1 1 c 3
x a c x a c (1 a )
2 2
z y x 1 a c 1 y
or a c 3 since
x t (1 a ) c 2 t
Thus the solution is

z x 1 a y c y t
,
x a (1 a ) t 3 t x

Self Assessment

1. Solve
x (y z) p + (y) (z x) q = z(x y)
2
2
2. x p + y q = z 2
3. p + q = z/a
2
4. zp zq = z + (x + y) 2
u u u
5. x y z xyz
x y z

6. tan x p + tan y q = tan z
17.4 Some Special Types of Equations

We have so far studied the method of solving the equations of the type
Pp + Qq = R.
Now, before we take up the general method of Charpit to solve the partial differential equations
of the first order but of any degree, we will deal with some special types of equations which can
be solved by methods other than the general method. We give here four simple standard forms
,, ,,
for which complete Integral can be obtained.

LOVELY PROFESSIONAL UNIVERSITY 253