Page 61 - DMTH202_BASIC_MATHEMATICS_II

Basic Mathematics-II

Notes
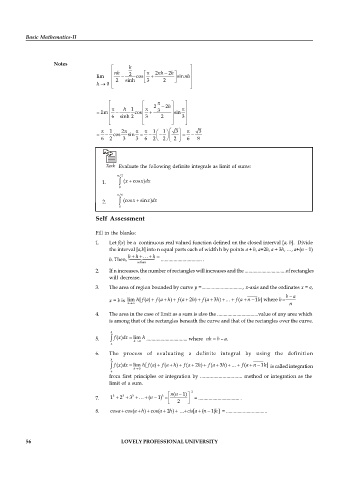
 h 
 nh 2   2nh  2h  
lim   cos    sinnh 
h  0  2 sinh  3 2  
 

    
  h 1  2 3  2h   
 lim   cos    sin 
 6 sinh 2  3 2  3 
     
 1 2   1  1   3   3
  cos sin         
6 2 3 3 6 2  2   2  6 8

Task Evaluate the following definite integrals as limit of sums:
 /2
x
1.  (x  cos )dx
0
 /6
x
2.  (cosx  sin )dx
0
Self Assessment

Fill in the blanks:
1. Let f(x) be a continuous real valued function defined on the closed interval [a, b]. Divide
the interval [a,b] into n equal parts each of width h by points a + h, a+2h, a + 3h, …, a+(n – 1)
h h   h 


h. Then, ................................ .
ntimes
2. If n increases, the number of rectangles will increases and the ................................of rectangles
will decrease.
3. The area of region bounded by curve y =................................, x-axis and the ordinates x = a,

b a
)

h

h
x = b is lim [ ( )h f a  ( f a h  ( f a  2 ) ( f a 3 )   ( f a n 1 ] where h
h
n n
4. The area in the case of limit as a sum is also the ................................value of any area which
is among that of the rectangles beneath the curve and that of the rectangles over the curve.
b
x
5.  f ( )dx  lim h ................................ where nh = b – a.
h 
0
a
6. The process of evaluating a definite integral by using the definition
b
a
f
h
h
)
x
h
h


 f ( )dx  lim [ ( )  ( f a h  ( f a  2 )  ( f a  3 )   ( f a n  1 ] is called integration
h  0
a
from first principles or integration by ................................ method or integration as the
limit of a sum.
 2
( n n 
1)
3 
3
3
3
7. 1  2  3    (n 1)    = ................................ .
 2 

8. cosa  cos(a h ) cos(a  2 )   cis [a (n 1) ]
h= ................................
h

56 LOVELY PROFESSIONAL UNIVERSITY